Professional Summary
About Me
I am working as a Visiting Assistant Professor of Mathematics at the Ramapo College of New Jersey. I received my Bachelor’s degree in Mathematics in 2015 from Ramjas College, University of Delhi, and a Master’s degree in Applied Mathematics in 2017 from the National Institute of Technology, Warangal, Telangana.
I earned my Ph.D. in Applied Mathematics in 2025 from the Department of Mathematical Sciences, New Jersey Institute of Technology, where I was advised by Dr. Roy Goodman.
Education
PhD Applied Mathematics, 2025
New Jersey Institute of Technology
MSc Applied Mathematics, 2017
National Institute of Technology, Warangal, India
BSc Honours Mathematics, 2015
Ramjas College, University of Delhi, India
Interests
Phase portraits and the bifurcation set for the three-vortex interaction system
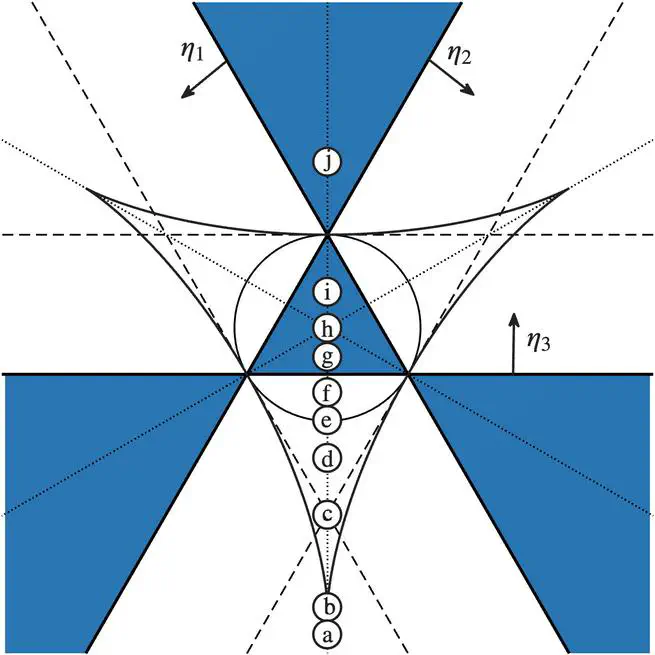
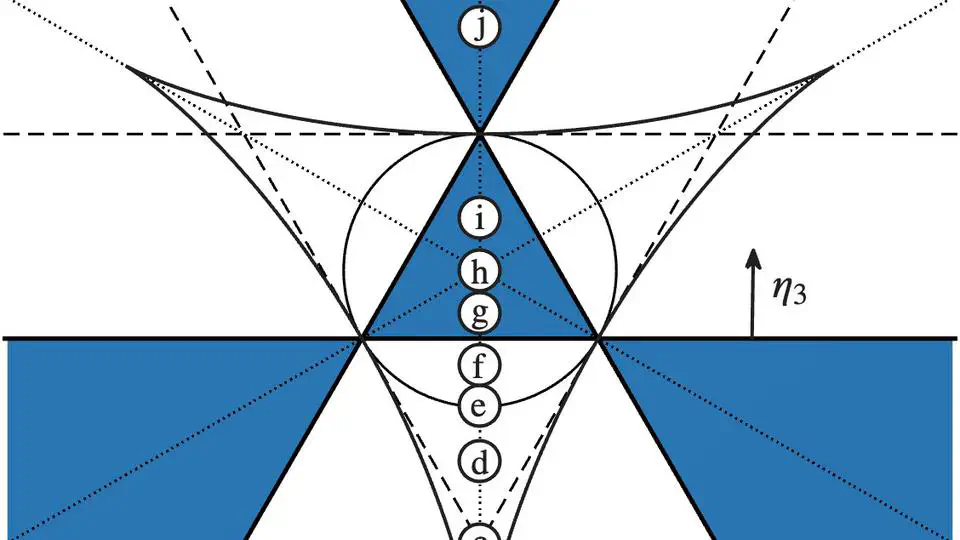
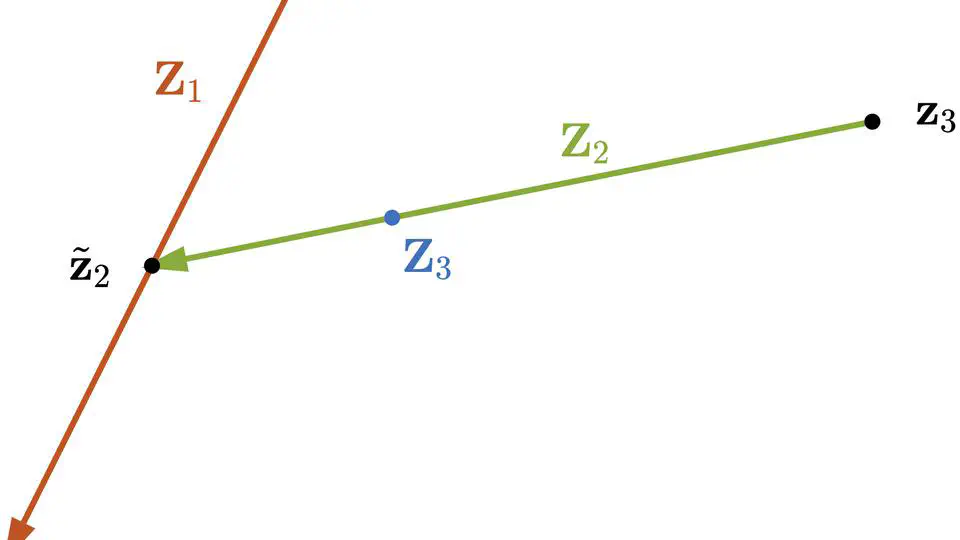
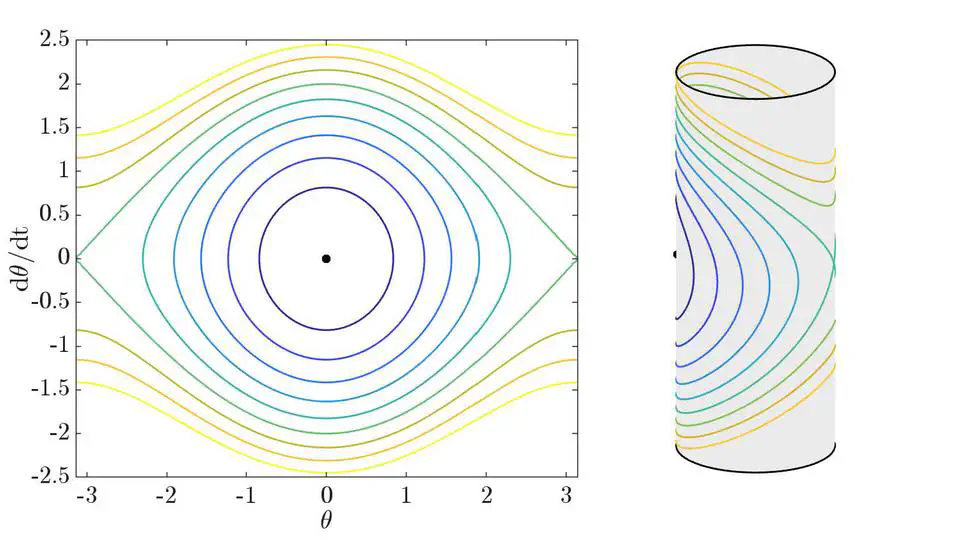
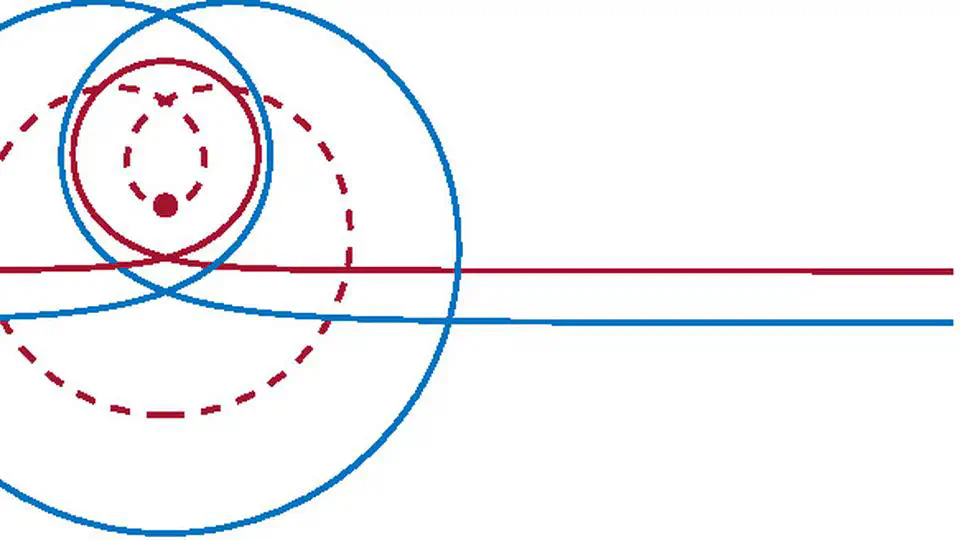
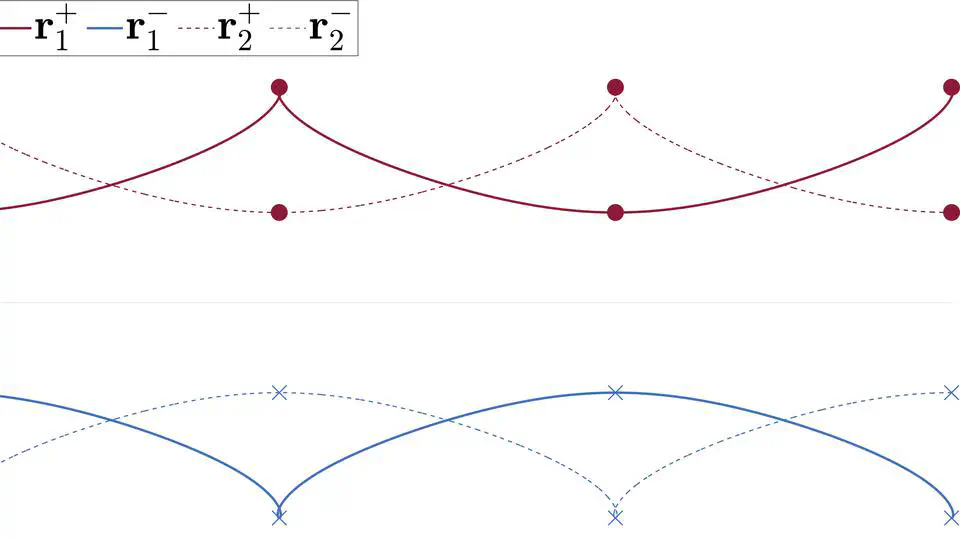
We derive a symplectic reduction of the evolution equations for a system of three point vortices and use the reduced system to succinctly explain a kind of bifurcation diagram that …
A new canonical reduction of three-vortex motion and its application to vortex-dipole scattering
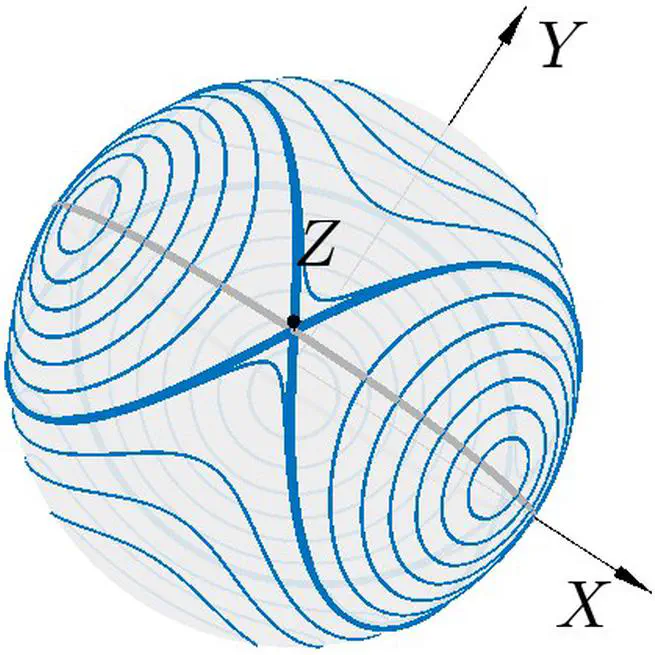
We use Jacobi coordinates and Nambu brackets to derive a new representation of the motion of three vortices which, unlinke all previous reductions, introduces no singularities into …
